The Zeros of the Partial Sums of ez
Roger Hui
Reference 26 of Ken Iverson’s Ph.D. thesis [0] was:
| Iverson, K.E., “The Zeros of the Partial Sums of ez ”, Mathematical Tables and Other Aids to Computation 7 (1953). |
I quickly found a citation [1] on the internet:
@Article{Iverson:1953:ZPS,
author = "K. E. Iverson",
title = "The Zeros of the Partial Sums of $e^z$",
journal = j-MATH-TABLES-OTHER-AIDS-COMPUT,
volume = "7",
number = "43",
pages = "165--168",
month = jul,
year = "1953",
CODEN = "MTTCAS",
ISSN = "0891-6837",
bibdate = "Tue Oct 13 08:06:19 MDT 1998",
bibsource = "http://www.math.utah.edu/pub/tex/bib/
mathcomp1950.bib; JSTOR database",
acknowledgement = ack-nhfb,
fjournal = "Mathematical Tables and Other Aids
to Computation",
}
From thence a helpful J Forum member provided a link to the actual text [2]. This is the earliest Ken Iverson publication I have found so far. The paper began thus:
|
The location of the zeros of certain entire functions
has received considerable attention in the literature,
and it was suggested by R.S. Varga that a table of
the zeros of certain truncated power series might
be of interest.
Accordingly, the zeros of the truncated exponential series
|
The table entry for n = 23 stated:
|
The verb p. in J [3] works with polynomials. The dyad x p. y evaluates the polynomial x at y ; the monad p. x converts x between coefficients c and multiplier and roots (m;r) . The coefficients of the partial sum of ez for n=23 are:
c=: % ! i.24 c 1 1 0.5 0.166667 0.0416667 0.00833333 0.00138889 ...
Converting from coefficients to muliplier and roots:
mr=: p. c
mr
┌───────────┬────────────────────────────────────────────────
│3.86817e_23│13.1749j10.1263 13.1749j_10.1263 7.72434j10.9536 ...
└───────────┴────────────────────────────────────────────────
r=: > {: mr
r
13.1749j10.1263 13.1749j_10.1263 7.72434j10.9536 ...
The roots from 1953 are ordered in increasing modulus, with the real root last; the roots computed by J are ordered in decreasing modulus. We compare the evaluation of the roots from 1953 and of the reordered roots computed by J:
r1953=: _7.192685907451j1.475059195082 ... _5 ]\ | c p. r1953 7.36698e_14 7.36698e_14 1.37043e_13 1.37043e_13 1.20857e_13 1.20857e_13 6.67108e_14 6.67108e_14 8.19945e_14 8.19945e_14 1.53714e_13 1.53714e_13 2.75251e_13 2.75251e_13 6.80251e_12 6.80251e_12 1.12317e_10 1.12317e_10 3.0922e_9 3.0922e_9 5.55512e_8 5.55512e_8 9.19177e_11 0 0 _5 ]\ | c p. 1 |. r /: | r 3.76597e_14 3.76597e_14 4.7101e_14 4.7101e_14 2.6361e_14 2.6361e_14 5.01272e_14 5.01272e_14 9.52945e_14 9.52945e_14 3.9363e_14 3.9363e_14 2.26531e_13 2.26531e_13 3.41104e_13 3.41104e_13 3.58005e_12 3.58005e_12 3.33604e_11 3.33604e_11 3.7143e_10 3.7143e_10 1.73195e_14 0 0
I survey the last results with considerable relief.
It would have been shameful to produce a worse result
59 years later.
References
| [0] | Iverson, K.E., Machine Solutions of Linear Differential Equations — Applications to a Dynamic Economic Model, Doctoral Thesis, Harvard University, 1954-01. http://www.jsoftware.com/papers/MSLDE.htm |
| [1] | http://ftp.math.utah.edu/pub//tex/bib/mathcomp1950.html#Iverson:1953:ZPS |
| [2] | http://www.ams.org/journals/mcom/1953-07-043/ S0025-5718-1953-0057013-0/S0025-5718-1953-0057013-0.pdf |
| [3] | Hui, R.K.W., and Iverson, K.E., J Introduction and Dictionary, Jsoftware Inc., 2012. http://www.jsoftware.com/help/dictionary/dpdot.htm |
| created: | 2012-08-14 07:25 |
| updated: | 2014-01-08 14:30 |
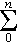 zk/k!
zk/k!